The Quantum Limits of Human Senses, and Other Cool-Sounding Crap

One of my favorite cool science facts was one I picked up years ago when reading about quantum physics.  After reading about how wondrous and strange quantum mechanics is, you then read how you’ll never actually see the strange effects in your day-to-day macroscopic world.  How disappointing to read about how reality is far more strange than you’d expect, then find out you have to go to great lengths to detect the weirdness – and forget about seeing it with your own unaided eyes.

Oh, except for your eyes – as it turns out, your eyeballs just might be sensitive enough to see A SINGLE FREAKING PHOTON.

Think about that – you can see an individual particle of light.  The smallest possible quantum unit in this sensory modality, and we can detect it unaided, with our eyes!  How’s that for quantum mechanics not being observable at the macroscopic level?  That got me thinking – could this also be true for the other senses?  How close can we get to the “quantum limit”, the smallest possible unit of detection, for our other senses?  Can any of the other sense get as close to the limit as eyesight?  Let’s take a tour of the limits of our senses, and find out.

Vision

Let’s start with vision – is it true that we can see a single photon?  How sensitive is eyesight?

The king in this area of research is William Bialek, a biophysics professor at Princeton University.   I’m sad to report he isn’t the father of Mayim Bialik (the different spelling of their names tipped me off, sleuth I am).  Biophysics is the hot new area in science now (especially for physicists trying to find some fertile areas of research that don’t involve a 50-mile-radius accelerator and 6,000 coauthors), but Dr. Bialek has been at it for decades.  His main area of work can be boiled down to asking the question: are our senses as good as they possibly could be?  If you work out the best possible hearing (for example) that a bag of saltwater and proteins could possibly do, obeying the laws of physics, how close are we to that limit?  It turns out that pretty much wherever he looked, Bialek found that we (and other organisms) are doing just about the best you could possibly do, without breaking the laws of physics.

The cutoff for light sensitivity is fuzzy – it’s not as though below 100 photons, the eye just claps its hands [FN:  its teeny eyeball hands] and says “I’m out”.  In reality fainter light flashes get harder and harder to call as real.  Eventually they get so faint that the signal is lost somewhere along the way, maybe in the eye, in the optic nerve, or even in the brain, and the flash of light never gets registered in our consciousness.  So how many photons need to be in that little puff of light to be reliably seen?  To find out, we need to explore the hilariously-named subdiscipline of psychophysics, the quantitative study of how our senses work.

Your eyes have their own “dark noise” that will look like very faint flashes of light, even in complete darkness – this phenomenon (awesomely called “Eigengrau”) comes from the inevitable random “false alarms” of the pigment molecule rhodopsin, the molecule that actually does the photon-catching.  When a photon hits a rhodopsin molecule in your retina, the molecule uses the absorbed energy to change its own shape, which is eventually converted to a nerve impulse that makes it to your brain.  Every once in a great while, a rhodopsin molecule will flop itself into the “photon detected” state purely by chance.  It doesn’t happen much, and is completely dwarfed by the normal amount of light coming in during yer normal daily activities, but in a completely darkened room it will look just like little flashes of dim light.

So we know that there’s an unavoidable faint “speckle” in our visual system caused by rhodopsin switches going off occasionally at random.  This ain’t going away – it’s a limit imposed essentially by physics, by the thermodynamics of the rhodopsin molecule.  And your brain ought to find a way to ignore it, otherwise you’d see a constant “visual snow” day and night, eyes open or closed.  In order to make this crap invisible, your brain needs to tune this out – but by tuning it out, it resigns itself to missing any real flashes of light that are just as faint.  So the theoretical upper limit to sensitivity ought to be juuust brighter than these spontaneous phantom “flashes”.

But how much worse do our eyes do?  If the upper limit to our vision sensitivity turned out to be the random fluttering of rhodopsin molecules, that would be pretty incredible.

Every scientific paper has a graph that looks exactly like this. It's precisely the same graph, recycled over and over again, and no one's noticed.

And, it’s true – experiments show that our eyes can reliably see a faint flash of light that is just barely above this background dark noise.  Amazingly enough, humans report they can see flashes of light consisting of less than 10 photons – maybe down to 2 or 3!  Just a single photon hitting one eyeball cell will trigger a neuron to fire, and the brain will declare success if it sees just a couple of those firings at about the same time.  There’s a cool picture in Bialek’s book Spikes (page 203, reproduced here) showing a recording from a rod cell in a toad as single photons were sent in.  You can see a little bump in the neuron’s voltage when one photon comes in, and occasionally see other little bumps happen spontaneously, presumably from a rhodopsin molecule going off by itself.  Since the brain can’t distinguish the random rhodopsin false-alarms from a real photon (they both look like a neuron firing, after all), it decides not to declare “I Seen Somethin” until it gets a couple of photons at the same time, a reasonable stance to take.

So let’s say you’re not satisfied with our eyeballs crapping out below ~3 photons – what can you do to improve it?  Well, the reason the rhodopsin molecule occasionally goes off all by itself is that it’s moving – all the molecules in your body are constantly moving & vibrating, which is what we call heat.  And the hotter we get, the more our molecules move around, and the more often the rhodopsin molecule will accidentally flop itself over to the “photon detected” state.  So it stands to reason, if you cooled yourself down, you’d settle down them feverish rhodopsins and thereby reduce the dark noise in your eyeballs.  So if you want to squeeze out another photon or two in sensitivity, or if your Eigengrau is bugging the shit out of you, just cool your eyeballs down to absolute zero and it’ll stop.

So let’s be a little conservative here and say the visual system needs to detect five photons to see something with good reliability.  Vision therefore can detect signals just five or so times the absolute lowest quantum limit.  Pretty impressive!

Human Hearing

The minimum detectable sound a human can hear is about 2 x 10^-5 pascals.  A “pascal” is a unit of pressure – and 2 x 10^-5 pascals is, scientifically speaking, “pretty damn faint”.  Air pressure is about 100 thousand pascals, to give you an idea.  When air pressure changes, like when you’re heading up the elevator to the top of the Sears tower, slight changes in atmospheric pressure are so dramatic to your sensitive eardrum that it hurts.  Your eardrum will *burst* at a pressure change of about 20 thousand pascals, so the lower limit of hearing is impressively small.

So what would be the hearing equivalent of the eye detecting a single photon?  Well, it would be your ears detecting just a single molecule of air moving.  Let’s say that the “quantum limit” for hearing is a single oxygen molecule (0₂) chugging along and bonking into your eardrum.  If we could hear a single particle of oxygen bonk against our eardrum, that would be just as impressive as seeing a single photon.

So, how fast would a single oxygen molecule need to be going to achieve this minimum pressure against our eardrum?  Using the old kinetic theory of gases result,

By my calculations, a single oxygen molecule would have to be going 3.35 x 10^10 meters per second to reach the minimum pressure detectable by the human eardrum.  That’s pretty damn fast.  In fact that’s about 100 times faster than the speed of light.  In fact, this oxygen molecule would have to be going almost warp 5, in the Star Trek speed units.

Putting aside the ugly reality that this speed is physically impossible to achieve, how much faster is this than our “quantum limit”?  Yer typical air molecule jetting around town at room temperature is going about 500 m / s.  So, the minimally-hearable oxygen molecule is going about 70 million times faster than the typical air molecule.

Ears are hard to draw

Let’s look at it another way.  In room-temperature air, each particle is going about 500 m / s (surprisingly fast, eh?), and there are a TON of them — almost 10²⁰ of them per cubic centimeter of air.  What’s the minimum number of them we could hear?  It turns out, if we gathered a bunch of oxygen molecules together and threw them at your eardrum at the typical speed of 500 m/s, we’d need a puff of about 10¹⁵ molecules per cubic centimeter.  Given that the eardrum is smaller than that, let’s shave off a couple orders of magnitude for the volume of air gently wafting against your eardrum, and estimate that we’d be able to hear at minimum 10¹¹ – 10¹² oxygen molecules — about a trillion of them are needed.  That sounds like a lot, but that’s still a LOT less than the density of air at room temp.

But room temperature is kinda fast — let’s go crazy and compare our Warp 5 oxygen molecule (exploring ears where no oxygen molecule has gone before) to the minimum possible velocity, set by the quantum limit of the uncertainty principle.  Let’s say we’re confining the oxygen molecule to a space about 10 millimeters long, about the size of an eardrum.  Then, by the uncertainty principle:

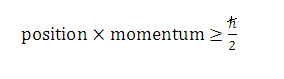the minimum possible velocity that an oxygen molecule could get down to in this small space is about 10^-7 meters per second.  That’s pretty damn slow.  So strictly speaking, the smallest possible “sound” we could hear would be an oxygen molecule going 10^-7 m/s, but you’d have to speed it up to 10⁺¹⁰ m/s to actually hear it.  That’s a factor of seventeen orders of magnitude above the theoretical quantum limit of hearing.  Not quite as impressive as vision, if I do say so myself.

While fun to compute, I think this is a little extreme — after all, we didn’t alter the energy of the photon hitting our eyeball, which would be the fair thing to do to compare to hearing to vision.  So let’s back off a bit and declare that human hearing is “only” a factor of about a trillion above the quantum limit.

The inner ear: simplicity incarnate

But before you write off hearing as the bastard child of the senses, check out the sensitivity of the hair cell, the little sensors inside your ears that actually do the hearing.  When you step inside the human inner ear, you’ll find tiny little hairs inside the cochlea, that are designed to wave in the breeze of sound waves – as they wave, the amount they get displaced is picked up by nerve impulses, which get sent to the brain.  As it turns out, these little hairs are extremely sensitive to faint breezes – our friend Bialek has estimated that your brain can detect when these little hair cells move by as little as 10^-10 meters – that’s an angstrom, to put it in perspective.  Wait, lemmie put it in more perspective – the brain can detect when these hair cells are displaced a SUBATOMIC DISTANCE!  That’s pretty damn small.  Yeah, it’s true that you won’t notice a single hair cell moving one angstrom (you have about 20,000 of them in your cochlea), and it’s true that the sound energy goes through a lot of steps to get from eardrum to hair cilia – so this is perhaps not quite as impressive as single-photon detection in the visual system, but it’s still pretty impressive.

Interestingly enough, the ears aren’t just passive funnels collecting sound waves to bounce off the hair cells.  To help improve sensitivity and beat down background noise, your ears are actually active listeners.  They use positive feedback to amplify signals coming in, thereby boosting your hearing (nanananananana…nananananaaaa…).  Sort of interesting in its own right, but the even cooler fact is that sensors that have positive feedback like this, sensors that are active not passive, will sometimes become unstable and do unpredictable things.  (Microphone feedback is an example of positive feedback going wild.)  What does our ear do in this situation?  It emits its own sound.  Called otoacoustic emissions, these sounds are actually created by your ear, as a byproduct of the mechanisms that amplify faint sounds.  Stick a microphone in your ear, and you’ll be able to hear them.  In fact people with hearing damage often have wound up damaging this active-feedback system in their ears, so their ears no longer make sounds – you can test for hearing damage by seeing if the telltale ear sounds have stopped.

If you’re in the mood to be freaked the hell out, check out Maryanne Amacher’s work on youtube – she was an experimental musician who composed works designed to stimulate these otoacoustic emissions in the audience.  Her music apparently evokes these sounds in your ears as you listen, making your own ears part of the performance.  Check out her “Synaptic Island” on youtube, and be sure to really crank it up your computer speakers in your work cubicle.

Smelling

So how little can we smell?  As you likely learned in junior high, smelling is simply little molecules of whatever you’re smelling wafting up into your nose, where they’re caught by olfactory receptors.  You likely followed this lesson about 5.4 minutes later with a horrible realization about bathrooms.  So if smelling were as badass as vision, we’d be able to pick up just a molecule or two of odor – that would be the equivalent “quantum limit” for odor.

Apparently Stuiver and de Vries demonstrated years ago that the human olfactory system can occasionally pick up a single molecule, but overall needs about 50 molecules for reliable smelling.  Apparently they worked this out back in the sixties, but weren’t nice enough to make their papers available on the net, so I don’t have a copy of their book to peruse myself to confirm this number.  But this seems a little funny to me – supposedly dogs have a sense of smell about a thousand times better than ours, suggesting that Fido ought to be able to smell about 5 percent of a single molecule.  Hmmm…

The awesomely named crotyl mercaptan has an odor threshold of about 0.3 parts per billion, the minimum concentration at which humans can reliably smell it.  This sounds pretty amazing, but it means there are close to a trillion molecules of odorant in the air we breathe in when we smell it.  (There are 10¹⁹ molecules in one cc of air, after all – so assuming we inhale a couple of cc’s when we smell, one part in a billion is still a lot of molecules.)  So something seems off here.

My suspicion, until I can get over to the library, put on the white gloves and peruse Stuiver’s work, is that the limit of ~50 molecules is per receptor – and since we have millions of receptors in our noses, the overall minimum amount of stuff we need for the smell to reach our consciousness is approaching a trillion molecules.  If we presume the stinkiest crud might reach an odor threshold of ~0.1 parts per billion, assuming we have ~10 million total receptors, and assuming we can get by with inhaling 1 cc of air to smell it, this gives us a theoretical limit of 13.4 billion molecules required, or 1.3 molecules per receptor – so it all hangs together.  It looks like each receptor is capable of picking up one molecule, but probably needs around 50 to reliably pick up the odor from background stench, and your nose needs all of the receptors to reach this minimum together in order for your brain to pick up the smell.  At minimum 10 or 20 billion molecules.  Wafting directly into your nose.  And that’s just for the barely perceptible odor – by the time a stench is overwhelmingly rank, you’ve got several orders of magnitude more molecules of the offender in your nose. ¹

"Hello ma'am, I'm here to pick up your daughter"

Researching the limits of smell led me to my next Christmas present for all my loved ones:  The Nasal Ranger, the most awesome technological breakthrough I’ve seen the last hour.  I’m not exactly sure what it does, but I like to imagine that it gives you super-smelling powers.   I also like to imagine how his head would explode if you could manage to push a beer fart directly into the intake of this thing.

We can therefore conclude the human sense of smell is operating at about 10 billion times the lowest possible quantum limit, interestingly a little better than hearing.  Still, its pretty impressive that each odor receptor can reliably pick up just a handful of molecules of the odorant.  (I love using the word “odorant”.)   The difference between smelling and seeing, then, is that for smell we need all the receptors to pick up a signal for the brain to detect the percept, whereas for vision just one of the receptors is enough.  (I adore using the word “whereas”.)

Taste

Much like smelling, I’m figuring the natural “quantum limit” for taste would be the ability to taste a single molecule of something.  How close can we get?  As it turns out, not that well.

Bitter taste is the one we’re most sensitive to, out of the five basic tastes.  Quinine is used as the standard for testing the taste threshold of bitterness, and it’s just detectable at about 8 micromolar concentration.  That sounds pretty faint, but I can tell already it’s nowhere near one molecule.  The bitterest substance known to science is denatonium, which apparently has a taste threshold of 0.05 parts per million.  Apparently if you get up to 10 parts per million, it becomes unbearably bitter, so bitter that its added to stuff like shampoo and antifreeze to dissuade people from drinking them.

Let’s assume you need just a smidge of fluid on your tongue to taste something, say 0.01 cubic centimeter.  (For comparison a single drop of water is about 0.05 cc’s).  This gives us about 3.3 x 10²⁰ molecules of solution we’re tasting.  For denatonium, then, we need to get about 17 trillion molecules on our tongue to just barely taste it.  That’s just sad, human-sense-of-taste – just sad.  There are about 1 million taste receptors on your tongue (about 100 each for your 10,000 taste buds), so each receptor cell needs to see about 17 million molecules of denatonium to make a call.  Weak!

So taste more or less ties with smell, operating at about 10 orders of magnitude (10 billion) above the quantum limit.

Touch

Now we get to the most controversial case of all — human touch.  We all just need some human touch, am I right folks?  You with me?  *Hand slap*

So how sensitive is human touch?  The king of the world of touch sensitivity is Dr. Mandayam Srinivasan and his lab at MIT, the “touch lab”.  Years ago he did a test where tiny tiny dots were etched onto glass plates and felt with fingertips.  Turns out, a little dot about the width of a printed period and about three microns high was still felt-able.  If he used a rough texture, not just a little dot, people could detect it at just 75 nanometers high.   In contrast, a DNA molecule is about 2 nanometers wide.  That’s pretty damn amazing.

So what would the quantum limit be?  Well, we could set the lower limit at the size of the smallest known particles, quarks.  Too bad for us that quarks, as far as we can tell, have zero size.  Quarks, electrons, and other elementary particles of their ilk are assumed in theories to have a radius of zero.  That may not be true, but no one has yet been able to experimentally determine if these particles have finite size.  So let’s back off from this limit a bit.  Instead, let’s call the limit one angstrom, which is exactly a tenth of a nanometer and is the radius of the typical smallish atom.  (Hydrogen has a radius of about half an angstrom.)²

So,it looks like human touch has a sensitivity of about three orders of mangitude above the quantum limit.  Not bad!  In fact, that puts it in second place behind vision.  Now its true that this test did use a macroscopically large patch of texture, not a single little dot — in order to feel something 75 nanometers tall, it needs to be a few millimeters across.  So technically speaking, we’re close to the quantum limit only in one dimension.  Furthermore, we needed it to be “texture”, not just one flat squat lump — apparently we need to feel repeated up-and-down deviations of about 75 nanometers to be felt reliably.  But hey, even one dimension performing at ~100 times the quantum limit is pretty damn impressive, so I’m gonna give the silver medal to touch.

Conclusions

Pretty cool, huh?  No?  Well, I admit this is one of  my dorkier articles.  But still, its interesting to see how when judged by this “quantum limit” idea, human hearing might actually be worse than smelling, and human vision might be doing just about as good as it possibly could, according to the laws of physics.  That’s pretty damn cool.

Footnotes:

1.   Remember that horrible realization about smelling in a bathroom?  Huh?  Do you rememember?

2.  Yes, strictly speaking atoms don’t have a fixed size, as their electron clouds are smeared out across space without a definite boundary.  But hey, work with me here.